Perpetual Options FAQ
Everything you need to know about perpetual options.
What are perpetual options?
Perpetual options are options that are perpetual, i.e. they never expire.
How are perpetual options settled?
Perpetual options are cash-settled. Settlement is instant and takes place within 1 second of closing a position.
Are perpetual options similar to perpetual futures?
Yes. It's possible to construct a perpetual option that mimics a perpetual future 1:1.
To do so, create a perpetual call options contract with a strike price of zero. The payoff function of this options contract will be identical to the payoff function of a perpetual futures contract, if they both share the same underlying asset.
Do perpetual options have funding?
Yes. Just like perpetual futures, perpetual options have funding. Holding a perpetual option is generally more valuable than holding a perpetual future, hence why the funding fee is often higher.
Who earns the funding on a perpetual option?
Just as with the perpetual future, the seller (short) will typically be the party that earns the funding. The only exception is when the funding rate is negative. In this case, the holder earns the funding.
How does the seller of a perpetual option earn money?
From the premium that they charge upfront, when selling the option, and from the funding that they accumulate hourly.
How does the buyer of a perpetual option earn money?
From an increase in the price of the option.
Can you trade perpetual options on leverage?
On Everstrike, perpetual options are fully collateralized (maximum leverage of 1).
Can you get liquidated while trading perpetual options?
Holders (longs) can get liquidated if they are unable to pay the hourly funding fee.
Sellers (shorts) can get liquidated if the price of the option they are selling exceeds their liquidation price.
The liquidation price for perpetual options sellers is calculated in a similar fashion to the liquidation price for perpetual futures sellers.
Are the strike prices of perpetual options dynamic?
On Everstrike, all strike prices are dynamic. They are pinned around the 100-hour EMA (exponential moving average) of the underlier. This keeps all contracts relevant in perpetuity.
Why trade perpetual options instead of perpetual futures?
Perpetual futures are delta-one instruments. Their payoff functions are linear, and do not incorporate volatility. Perpetual options provide exposure to volatility, and the ability to trade on leverage with (minimal) risk of liquidation.
Perpetual options can represent a potentially infinite amount of payoff functions, while the perpetual future is limited to just two payoff functions (one for shorts, one for longs).
Why trade perpetual options instead of vanilla options?
For the same reason that traders trade perpetual futures instead of vanilla futures. To not have to concern themselves with expiration.
How are perpetual options priced?
There are multiple ways to price a perpetual option. The most popular way is to use a simple permutation of the Black Scholes formula:
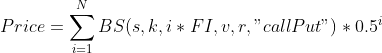
To use the formula, you'll need the Funding Period (10 hours, or 0.0011 years, on Everstrike), your volatility estimate (annualized volatility), an annual risk-free interest rate, and the number N (you can choose any N - however, higher N's will provide a more accurate price, at the expense of making the formula more computationally intensive - it is recommended to use a N of at least 10).
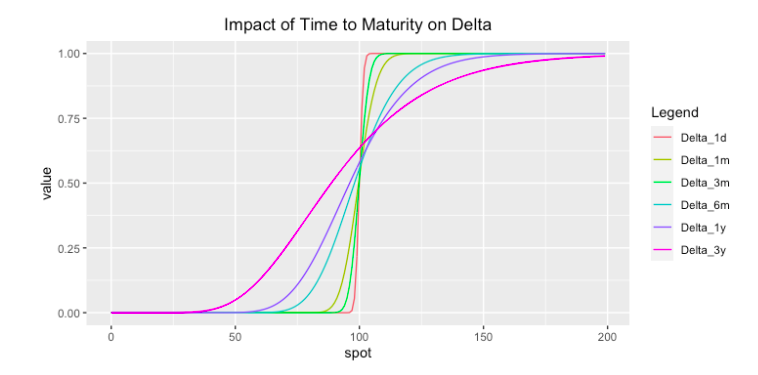
How are the greeks (i.e. delta) and the IV (implied volatility) of a perpetual option calculated?
The greeks (delta, gamma and vega) can be calculated in a way similar to the price. Just substitute the Black Scholes formula in the equation above with the formula for the greek that you are calculating (i.e. delta).
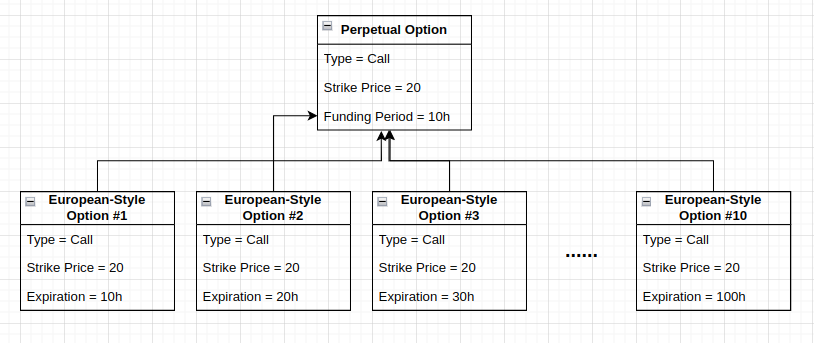
Can you trade perpetual multi-leg strategies, such as perpetual straddles?
It's possible to combine multiple perpetual options contracts, each with a different strike price, to create complex structures such as the straddle. Everstrike makes it easy to do so.
What is funding?
A periodic exchange of money between longs and shorts.
What is the funding rate?
The funding rate determines the size of the funding payment that is exchanged. It is expressed as a percentage of position size. If the funding rate is negative, shorts pay longs. Otherwise, longs pay shorts.
How often does funding take place?
On Everstrike, funding takes place once every hour (funding interval of 1 hour).
What impact does the funding interval have on the options price?
The shorter the funding interval, the greater the 'shortsightedness' of the option. Perpetual options with a funding interval of 1 hour (such as on Everstrike) are meant to recreate 0DTE vanilla options.
You can calculate the approximate expiration of an equivalent vanilla option by multiplying the TTNF (Time Till Next Funding) by twenty.
What is the funding period?
The minimum time to exchange a funding amount that sums to 100%.
Since the maximum hourly funding rate on Everstrike is 10%, it takes a minimum of 10 hours to exchange funding that sums to 100%. Hence, the funding period on Everstrike is 10 hours.
The funding period is used when calculating the funding rate, when using the Black Scholes formula to calculate the price of a perpetual option, and when calculating the delta, gamma and vega of a perpetual option.
The formulas used for the funding rate calculation can be found here.
What is the underlier?
The underlying asset of the perpetual option, i.e. BTC.
What is the underlier EMA?
The EMA (exponential moving average) of the price of the underlier. It's used for strike price calculation and for drift calculation.
What is drift?
The degree to which changes in the underlier EMA affects the intrinsic value of a perpetual option.
A drift of 1% per hour indicates that the underlier EMA causes the intrinsic value of the option to grow by 1% per hour.
Drift and funding are often correlated (contracts that have negative drift will often also have negative funding).
What is the index price of a perpetual option?
The option's intrinsic value. If the intrinsic value is negative, the index price defaults to 0.004% of the underlier.
What is the theoretical price of a perpetual option?
The price of the option, if calculated using a simple permutation of the Black Scholes formula:
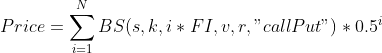
For more information, check out the pricing section of this article.
What is the mark price of a perpetual option?
The mark price is simply the average mid price of the order book (bid+(ask-bid)/2) within the last 30 seconds, scaled to fit within a certain price interval. The theoretical value of the option is used to determine this price interval.
Do perpetual options (generally) become more valuable with time?
It depends on the price performance of the underlier.
If the price of the underlier increases, the price of each option associated with the underlier will also increase.
If the price of the underlier decreases, the opposite will happen.
Most assets increase in value over time, making perpetual options (generally) increasing in value as well.
What is the TTNF (Time Till Next Funding) of a perpetual option?
The time until the contract's next funding exchange. TTNF is a proxy for expiration and is used to calculate the option's delta, gamma and vega.
What do I need to know about the gamma and vega of perpetual options?
Low TTNF = Low vega and high gamma (generally speaking).
High TTNF = High vega and low gamma (generally speaking).
Perpetual options on Everstrike have a low TTNF (maximum 1 hour), making them poor on vega and rich on gamma.
Where can I learn more about perpetual options?
Check out our blog posts:

Also check out the original whitepaper on Everlasting Options by Dave White, which inspired Everstrike's perpetual options implementation:

Where can I see the specifications for perpetual options on Everstrike?
You can see the specifications in the official docs: